Jacob
What goes around comes around.
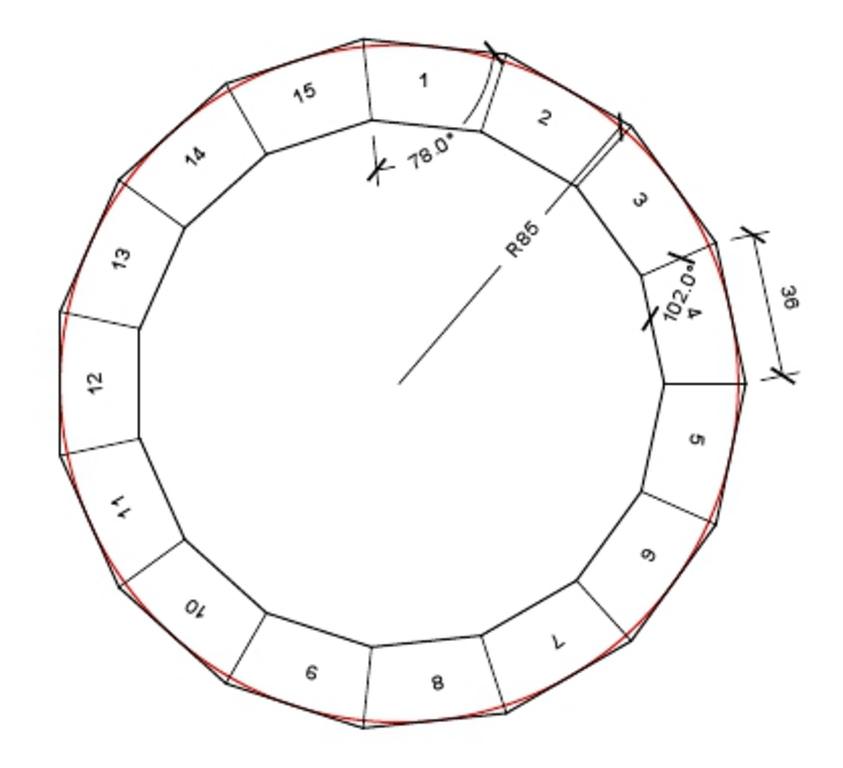
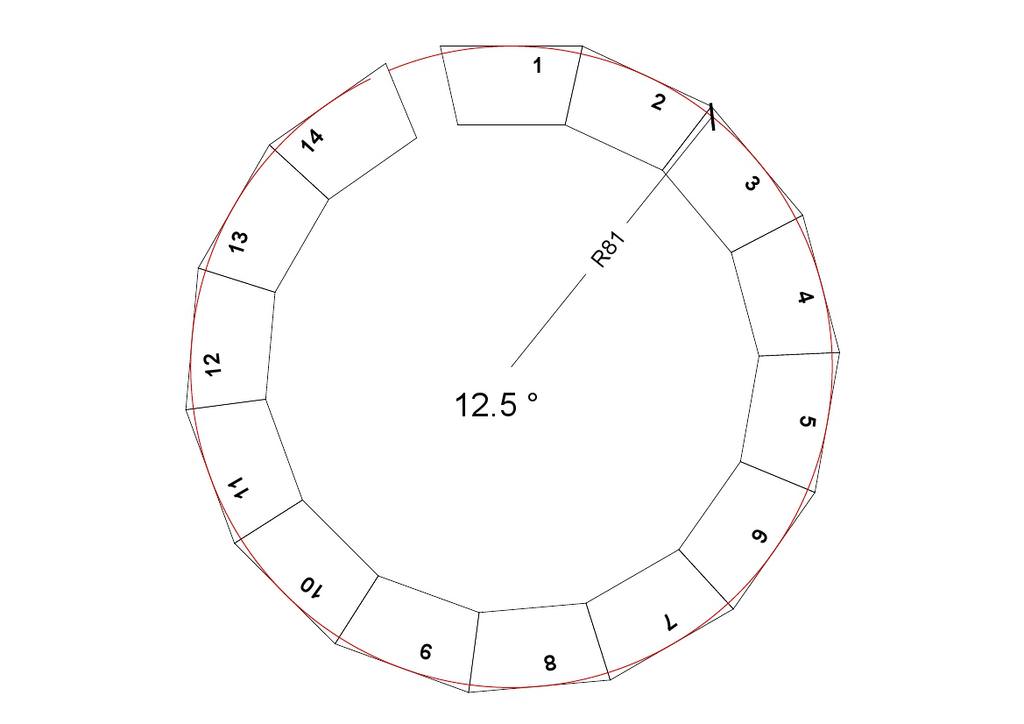
Also wrong.dizjasta":1uwq3b4r said:outer circumference of tub is 534.0 mm. Outside width of each stave is 35.6 mm.
35.6 would be the length of the arc of the circle of the outside face, if the staves are to be shaped to the circle, but the width of the staves would be the chord.
So calculation and SketchUp both get it wrong!
Stick with paper and pencil.